WnioskowanieStatystyczne/Analiza wariancji: Różnice pomiędzy wersjami
| (Nie pokazano 12 pośrednich wersji utworzonych przez tego samego użytkownika) | |||
| Linia 14: | Linia 14: | ||
posiada rozkład <math>F</math> z <math>f_{1}</math> i | posiada rozkład <math>F</math> z <math>f_{1}</math> i | ||
| − | <math>f_{2}</math> stopniami swobody | + | <math>f_{2}</math> stopniami swobody o wartości oczekiwanej <math>E(f)=\frac{f_{2}}{(f_{2}-2)}</math> |
<math> | <math> | ||
| Linia 43: | Linia 43: | ||
<math>f_{x}</math> stopniach swobody. | <math>f_{x}</math> stopniach swobody. | ||
| − | == | + | ==Analiza wariancji ''(ANalysis of VAriance — ANOVA)''== |
| − | |||
| − | |||
| − | + | <math>N</math> obserwacji <math>\{x_{i}\}_{i=1..N}</math> | |
| − | + | [[Plik:Anova1.png|500px]] | |
| + | podzielonych na <math>k</math> grup wedle jakiegoś kryterium: | ||
| + | <math>N=n_{1}+n_{2}+...+n_{k}</math>. | ||
| − | + | [[Plik:Anova2.png|500px]] | |
| − | |||
| − | |||
| − | + | Średnie wewnątrz grup | |
| − | |||
| − | |||
<math> | <math> | ||
\overline{x}_{i}=\frac{1}{n_{i}}\underset{j=1}{\overset{n_{i}}{\sum }}x_{ij} | \overline{x}_{i}=\frac{1}{n_{i}}\underset{j=1}{\overset{n_{i}}{\sum }}x_{ij} | ||
</math> | </math> | ||
| + | |||
| + | [[Plik:Anova3.png|500px]] | ||
| + | |||
| + | Średnia globalna <math>\overline{x}</math> | ||
| + | |||
| + | [[Plik:Anova4.png|500px]] | ||
Rozważmy sumę kwadratów odchyleń wszystkich elementów próby od | Rozważmy sumę kwadratów odchyleń wszystkich elementów próby od | ||
wartości średniej całej próby: | wartości średniej całej próby: | ||
| + | |||
| + | [[Plik:Anova5.png|500px]] | ||
| + | |||
<math>\begin{matrix} | <math>\begin{matrix} | ||
| Linia 78: | Linia 83: | ||
_{i})(\overline{x}_{i}-\overline{x}) | _{i})(\overline{x}_{i}-\overline{x}) | ||
\end{matrix}</math> | \end{matrix}</math> | ||
| + | |||
| + | |||
<math> | <math> | ||
| Linia 85: | Linia 92: | ||
n_{i}}{\sum }}(x_{ij}-\overline{x}_{i})=0 | n_{i}}{\sum }}(x_{ij}-\overline{x}_{i})=0 | ||
</math> | </math> | ||
| + | |||
<math> | <math> | ||
\underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }}( | \underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }}( | ||
\overline{x}_{i}-\overline{x})^{2}=\underset{i=1}{\overset{k}{\sum }}n_{i}( | \overline{x}_{i}-\overline{x})^{2}=\underset{i=1}{\overset{k}{\sum }}n_{i}( | ||
| − | \overline{x}_{i}-\overline{x}) | + | \overline{x}_{i}-\overline{x})^2 |
</math> | </math> | ||
| + | |||
| + | |||
<math> | <math> | ||
\underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }} | \underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }} | ||
| − | (x_{ij}-\overline{x | + | (x_{ij}-\overline{x})^{2}=\underset{i=1}{\overset{k}{\sum }}\underset{j=1 |
}{\overset{n_{i}}{\sum }}(x_{ij}-\overline{x}_{i})^{2}+\underset{i=1}{ | }{\overset{n_{i}}{\sum }}(x_{ij}-\overline{x}_{i})^{2}+\underset{i=1}{ | ||
\overset{k}{\sum }}n_{i}(\overline{x}_{i}-\overline{x} | \overset{k}{\sum }}n_{i}(\overline{x}_{i}-\overline{x} | ||
| − | ) | + | )^{2} |
</math> | </math> | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | inaczej | ||
| + | |||
| + | <math>\underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }} | ||
| + | (x_{ij}-\overline{x})^{2} = s_{wew}^{2}+s_{pom}^{2} | ||
| + | </math> | ||
| + | |||
Jeśli wszystkie pomiary pochodzą z tej samej populacji o wariancji | Jeśli wszystkie pomiary pochodzą z tej samej populacji o wariancji | ||
| Linia 104: | Linia 124: | ||
<math> | <math> | ||
| − | \frac{ | + | \frac{s^2_{wew}}{\sigma ^{2}}\ i\ \ \frac{s^2_{pom}}{\sigma ^{2}} |
</math> | </math> | ||
| Linia 111: | Linia 131: | ||
<math> | <math> | ||
| − | \frac{\left( n-k\right) | + | \frac{\left( n-k\right) s^2_{pom}}{\left( k-1\right) |
| − | + | s^2_{wew}} | |
</math> | </math> | ||
| Linia 132: | Linia 152: | ||
są nieobciążonymi estymatami wariancji populacji. | są nieobciążonymi estymatami wariancji populacji. | ||
| + | |||
| + | =Testy par a posteriori= | ||
| + | |||
| + | Testowanie ''a posteriori'' (inaczej ''post hoc'') -> [[WnioskowanieStatystyczne/Bonferroni | porównania wielokrotne]] | ||
Wersja z 18:08, 5 maj 2024
Wnioskowanie_Statystyczne_-_wykład
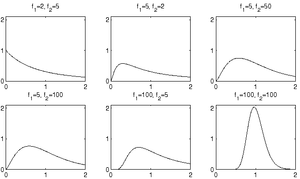
Rozkład F
Niech zmienne [math]x[/math] i [math]y[/math] mają rozkłady [math]\chi ^{2}[/math] o odpowiednio [math]f_{1}[/math] i [math]f_{2}[/math] stopniach swobody. Zmienna
[math] F=\frac{\frac{1}{f_{1}} x}{\frac{1}{f_{2}}y}=\frac{f_{2}x}{f_{1}y} [/math]
posiada rozkład [math]F[/math] z [math]f_{1}[/math] i [math]f_{2}[/math] stopniami swobody o wartości oczekiwanej [math]E(f)=\frac{f_{2}}{(f_{2}-2)}[/math]
[math] f(F)=\left( \frac{f_{1}}{f_{2}}\right) ^{\frac{f_{1}}{2}}\frac{\Gamma \left( \frac{1}{2}\left( f_{1}+f_{2}\right) \right) }{\Gamma \left( \frac{f_{1}}{2} \right) \Gamma \left( \frac{f_{2}}{2}\right) }F^{\frac{f_{2}}{2}-1}\left( 1+ \frac{f_{1}}{f_{2}}F\right) ^{-\frac{f_{1}+f_{2}}{2}} [/math]
Dla próby z rozkładu normalnego wielkość
[math] \chi ^{2}=\underset{i=1}{\overset{N}{\sum }}\frac{(x_{i}-\overline{x})^{2}}{ \sigma ^{2}} [/math]
podlega rozkładowi [math]\chi ^{2}[/math] o [math]f=N-1[/math] stopniach swobody. Jeśli dwie takie próby zostały pobrane z jednej populacji, to iloraz
[math] F=\frac{\left( N_{y}-1\right) \underset{i=1}{\overset{N}{\sum (}}x_{i}- \overline{x})^{2}}{\left( N_{x}-1\right) \underset{i=1}{\overset{N}{\sum }} (y_{i}-\overline{y})^{2}} [/math]
podlega rozkładowi [math]F[/math] o [math]f_{y}[/math] i [math]f_{x}[/math] stopniach swobody.
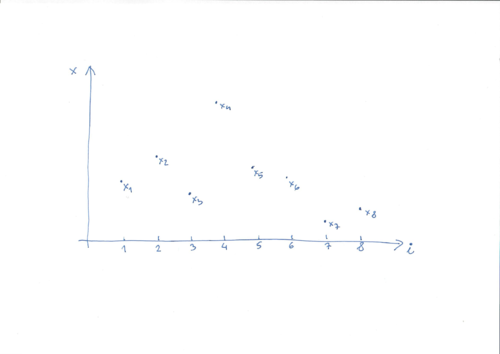
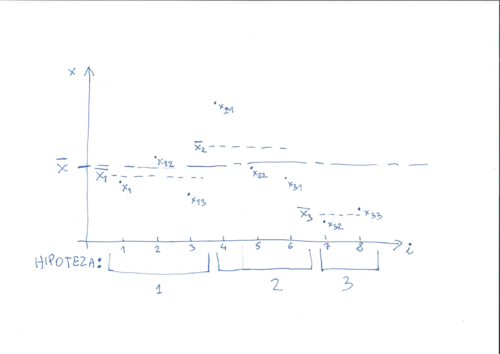
Analiza wariancji (ANalysis of VAriance — ANOVA)
[math]N[/math] obserwacji [math]\{x_{i}\}_{i=1..N}[/math]
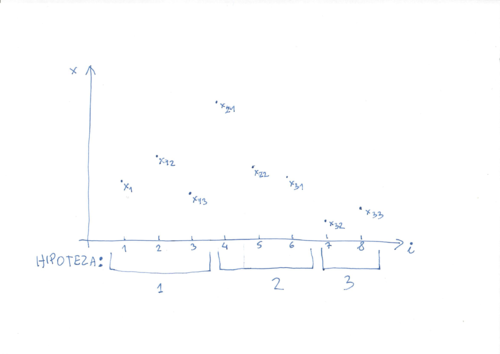
podzielonych na [math]k[/math] grup wedle jakiegoś kryterium: [math]N=n_{1}+n_{2}+...+n_{k}[/math].
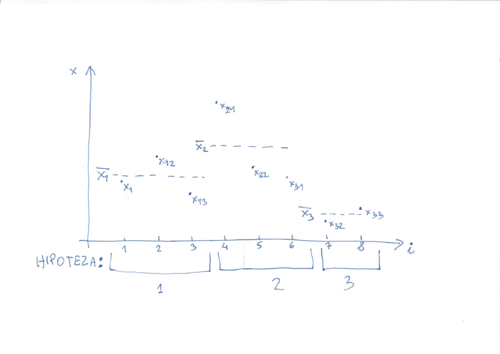
Średnie wewnątrz grup
[math] \overline{x}_{i}=\frac{1}{n_{i}}\underset{j=1}{\overset{n_{i}}{\sum }}x_{ij} [/math]
Średnia globalna [math]\overline{x}[/math]
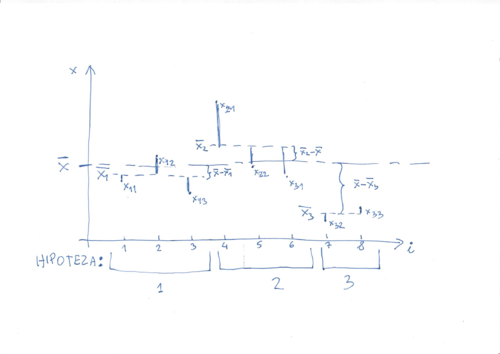
Rozważmy sumę kwadratów odchyleń wszystkich elementów próby od wartości średniej całej próby:
[math]\begin{matrix}
\underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }}
(x_{ij}-\overline{x})^{2}=\underset{i=1}{\overset{k}{\sum }}\underset{j=1}{
\overset{n_{i}}{\sum }}(x_{ij}-\overline{x}_{i}+\overline{x}_{i}-\overline{x}
)^{2}=\\
=\underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }}
(x_{ij}-\overline{x}_{i})^{2}+\underset{i=1}{\overset{k}{\sum }}\underset{j=1
}{\overset{n_{i}}{\sum }}(\overline{x}_{i}-\overline{x})^{2}+2\underset{i=1}{
\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }}(x_{ij}-\overline{x}
_{i})(\overline{x}_{i}-\overline{x})
\end{matrix}[/math]
[math] \underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }} (x_{ij}-\overline{x}_{i})(\overline{x}_{i}-\overline{x})=\underset{i=1}{ \overset{k}{\sum }}(\overline{x}_{i}-\overline{x})\underset{j=1}{\overset{ n_{i}}{\sum }}(x_{ij}-\overline{x}_{i})=0 [/math]
[math]
\underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }}(
\overline{x}_{i}-\overline{x})^{2}=\underset{i=1}{\overset{k}{\sum }}n_{i}(
\overline{x}_{i}-\overline{x})^2
[/math]
[math] \underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }} (x_{ij}-\overline{x})^{2}=\underset{i=1}{\overset{k}{\sum }}\underset{j=1 }{\overset{n_{i}}{\sum }}(x_{ij}-\overline{x}_{i})^{2}+\underset{i=1}{ \overset{k}{\sum }}n_{i}(\overline{x}_{i}-\overline{x} )^{2} [/math]
inaczej
[math]\underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i}}{\sum }} (x_{ij}-\overline{x})^{2} = s_{wew}^{2}+s_{pom}^{2} [/math]
Jeśli wszystkie pomiary pochodzą z tej samej populacji o wariancji
[math] \sigma ^{2}[/math], to
[math] \frac{s^2_{wew}}{\sigma ^{2}}\ i\ \ \frac{s^2_{pom}}{\sigma ^{2}} [/math]
podlegają rozkładom [math]\chi ^{2}[/math] o odpowiednio [math]n-k[/math] i [math]k-1[/math] stopniach swobody. Iloraz
[math] \frac{\left( n-k\right) s^2_{pom}}{\left( k-1\right) s^2_{wew}} [/math]
podlega rozkładowi [math]F[/math] o [math]k-1[/math] i [math]n-k[/math] stopniach swobody. Wyrażenia
[math] \frac{1}{n-k}\underset{i=1}{\overset{k}{\sum }}\underset{j=1}{\overset{n_{i} }{\sum }}(x_{ij}-\overline{x}_{i})^{2}\ oraz\ \ \frac{1}{k-1}\underset{i=1}{ \overset{k}{\sum }}n_{i}(\overline{x}_{i}-\overline{x})^{2} [/math]
czyli
[math] \frac{s_{wew}^{2}}{n-k}\ \ oraz\ \ \frac{s_{pom}^{2}}{k-1} [/math]
są nieobciążonymi estymatami wariancji populacji.
Testy par a posteriori
Testowanie a posteriori (inaczej post hoc) -> porównania wielokrotne